Primov algoritem

V tej vadnici boste izvedeli, kako deluje Primov algoritem. Prav tako boste našli delovne primere Prim-ovega algoritma v jeziku C, C ++, Java in Python.

Primov algoritem je minimalni drevesni algoritem, ki vzame graf kot vhod in najde podskupino robov tega grafa, ki

tvorijo drevo, ki vključuje vsako oglišče
ima najmanjšo vsoto uteži med vsemi drevesi, ki jih lahko oblikujemo iz grafa

Kako deluje Primov algoritem

Spada v razred algoritmov, imenovanih pohlepni algoritmi, ki najdejo lokalni optimum v upanju, da bodo našli globalni optimum.

Izhajamo iz ene točke in dodajamo robove z najmanjšo težo, dokler ne dosežemo cilja.

Koraki za izvajanje Primovega algoritma so naslednji:

Inicializirajte minimalno razpeta drevesa z naključno izbrano točko.
Poiščite vse robove, ki drevo povezujejo z novimi točkami, poiščite minimum in ga dodajte drevesu
Ponavljajte 2. korak, dokler ne dobimo minimalnega drevesa

Primer Primovega algoritma

Začnite s tehtano graf

Izberite vertex

Izberite najkrajšo rob iz te tocke in dodali, da

Izberite najbližjo vrhom še ni v raztopini

Izberite najbližjo rob še ni rešitev, če obstaja več možnosti, izberite eno naključno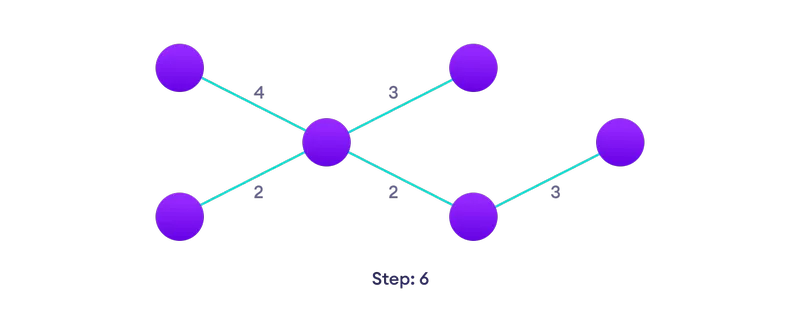

ponavljajte, dokler vas imeti raztegnjeno drevo

Psevkodo Primov algoritem

Psevkodo za algoritem prim kaže, kako ustvarimo dva niza točk U in VU. U vsebuje seznam točk, ki so bile obiskane, VU pa točko, ki jih ni. Eden za drugim premaknemo oglišča iz nastavljenega VU v U, tako da povežemo rob najmanjše teže.

 T = ∅; U = ( 1 ); while (U ≠ V) let (u, v) be the lowest cost edge such that u ∈ U and v ∈ V - U; T = T ∪ ((u, v)) U = U ∪ (v)

Primeri Python, Java in C / C ++

Čeprav se uporablja matrična predstavitev grafov sosednosti, lahko ta algoritem za izboljšanje njegove učinkovitosti uporabimo tudi s pomočjo seznama adjacency List.

Python Java C C ++

 # Prim's Algorithm in Python INF = 9999999 # number of vertices in graph V = 5 # create a 2d array of size 5x5 # for adjacency matrix to represent graph G = ((0, 9, 75, 0, 0), (9, 0, 95, 19, 42), (75, 95, 0, 51, 66), (0, 19, 51, 0, 31), (0, 42, 66, 31, 0)) # create a array to track selected vertex # selected will become true otherwise false selected = (0, 0, 0, 0, 0) # set number of edge to 0 no_edge = 0 # the number of egde in minimum spanning tree will be # always less than(V - 1), where V is number of vertices in # graph # choose 0th vertex and make it true selected(0) = True # print for edge and weight print("Edge : Weight") while (no_edge  G(i)(j): minimum = G(i)(j) x = i y = j print(str(x) + "-" + str(y) + ":" + str(G(x)(y))) selected(y) = True no_edge += 1

 // Prim's Algorithm in Java import java.util.Arrays; class PGraph ( public void Prim(int G()(), int V) ( int INF = 9999999; int no_edge; // number of edge // create a array to track selected vertex // selected will become true otherwise false boolean() selected = new boolean(V); // set selected false initially Arrays.fill(selected, false); // set number of edge to 0 no_edge = 0; // the number of egde in minimum spanning tree will be // always less than (V -1), where V is number of vertices in // graph // choose 0th vertex and make it true selected(0) = true; // print for edge and weight System.out.println("Edge : Weight"); while (no_edge < V - 1) ( // For every vertex in the set S, find the all adjacent vertices // , calculate the distance from the vertex selected at step 1. // if the vertex is already in the set S, discard it otherwise // choose another vertex nearest to selected vertex at step 1. int min = INF; int x = 0; // row number int y = 0; // col number for (int i = 0; i < V; i++) ( if (selected(i) == true) ( for (int j = 0; j G(i)(j)) ( min = G(i)(j); x = i; y = j; ) ) ) ) ) System.out.println(x + " - " + y + " : " + G(x)(y)); selected(y) = true; no_edge++; ) ) public static void main(String() args) ( PGraph g = new PGraph(); // number of vertices in grapj int V = 5; // create a 2d array of size 5x5 // for adjacency matrix to represent graph int()() G = ( ( 0, 9, 75, 0, 0 ), ( 9, 0, 95, 19, 42 ), ( 75, 95, 0, 51, 66 ), ( 0, 19, 51, 0, 31 ), ( 0, 42, 66, 31, 0 ) ); g.Prim(G, V); ) )

 // Prim's Algorithm in C #include #include #define INF 9999999 // number of vertices in graph #define V 5 // create a 2d array of size 5x5 //for adjacency matrix to represent graph int G(V)(V) = ( (0, 9, 75, 0, 0), (9, 0, 95, 19, 42), (75, 95, 0, 51, 66), (0, 19, 51, 0, 31), (0, 42, 66, 31, 0)); int main() ( int no_edge; // number of edge // create a array to track selected vertex // selected will become true otherwise false int selected(V); // set selected false initially memset(selected, false, sizeof(selected)); // set number of edge to 0 no_edge = 0; // the number of egde in minimum spanning tree will be // always less than (V -1), where V is number of vertices in //graph // choose 0th vertex and make it true selected(0) = true; int x; // row number int y; // col number // print for edge and weight printf("Edge : Weight"); while (no_edge < V - 1) ( //For every vertex in the set S, find the all adjacent vertices // , calculate the distance from the vertex selected at step 1. // if the vertex is already in the set S, discard it otherwise //choose another vertex nearest to selected vertex at step 1. int min = INF; x = 0; y = 0; for (int i = 0; i < V; i++) ( if (selected(i)) ( for (int j = 0; j G(i)(j)) ( min = G(i)(j); x = i; y = j; ) ) ) ) ) printf("%d - %d : %d", x, y, G(x)(y)); selected(y) = true; no_edge++; ) return 0; )

 // Prim's Algorithm in C++ #include #include using namespace std; #define INF 9999999 // number of vertices in grapj #define V 5 // create a 2d array of size 5x5 //for adjacency matrix to represent graph int G(V)(V) = ( (0, 9, 75, 0, 0), (9, 0, 95, 19, 42), (75, 95, 0, 51, 66), (0, 19, 51, 0, 31), (0, 42, 66, 31, 0)); int main() ( int no_edge; // number of edge // create a array to track selected vertex // selected will become true otherwise false int selected(V); // set selected false initially memset(selected, false, sizeof(selected)); // set number of edge to 0 no_edge = 0; // the number of egde in minimum spanning tree will be // always less than (V -1), where V is number of vertices in //graph // choose 0th vertex and make it true selected(0) = true; int x; // row number int y; // col number // print for edge and weight cout << "Edge" << " : " << "Weight"; cout << endl; while (no_edge < V - 1) ( //For every vertex in the set S, find the all adjacent vertices // , calculate the distance from the vertex selected at step 1. // if the vertex is already in the set S, discard it otherwise //choose another vertex nearest to selected vertex at step 1. int min = INF; x = 0; y = 0; for (int i = 0; i < V; i++) ( if (selected(i)) ( for (int j = 0; j G(i)(j)) ( min = G(i)(j); x = i; y = j; ) ) ) ) ) cout << x << " - " << y << " : " << G(x)(y); cout << endl; selected(y) = true; no_edge++; ) return 0; )

Primov vs Kruskalov algoritem

Kruskalov algoritem je še en priljubljen algoritem zajemajočega drevesa, ki uporablja drugačno logiko za iskanje MST grafa. Namesto da začne iz oglišča, Kruskalov algoritem razvrsti vse robove od majhne do visoke in nenehno dodaja najnižje robove, pri čemer ignorira tiste robove, ki ustvarjajo cikel.